Follow-Up: The Infinite Series and the Mind-Blowing Result

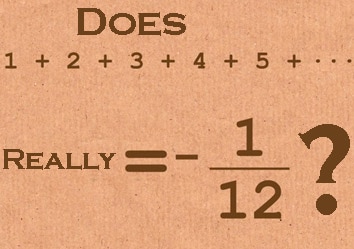
Yesterday, I posted an article about a math video that showed how you can sum up an infinite series of numbers to get a result of, weirdly enough, -1/12.

A lot of stuff happened after I posted it. Some people were blown away by it, and others ⦠not so much. A handful of mathematicians were less than happy with what I wrote, and even more were less than happy with the video. I got a few emails, a lot of tweets, and some very interesting conversations out of it.

I decided to write a follow-up post because I try to correct errors when I make them and shine more light on a problem if it needs it. There are multiple pathways to take here (which is ironic because thatâs actually part of the problem with the math). Therefore this post is part 1) update, 2) correction, and 3) mea culpa, with a defense (hopefully without being defensive).

Let me take a moment to explain right away. No, there is too much. Let me sum up^*:

1) The infinite series in the video (1+2+3+4+5+â¦) can in fact be tackled using a rigorous mathematical method and can in fact be assigned a value of -1/12! This method is quite real and very useful. And yes, the weirdness of it is brain melting.

2) The method used in the video to write out some series and manipulate them algebraically is actually not a great way to figure this problem out. It uses a trick thatâs against the rules, so strictly speaking it doesnât work. Itâs a nice demo to show some fun things, but its utility is questionable at best.

3) I had my suspicions about the method used in the video but suppressed them. That was a mistake.

Thatâs the tl;dr version. Hereâs the detail.

1) Terms of Endearment

In math, you have to set up rules that allow you to do whatever it is you want to do. These rules can be self-consistent, totally logical, and very useful. Or, they can be self-consistent, totally logical, and not useful. Let me give you an example, inspired by a conversation I had with the delightful mathematician Jordan Ellenberg, who contacted Slate and me after my article went up.

Imagine you come upon a society that uses numbers only as integer magnitudes, that is, to measure the amount of something in integer units (1, 2, 3, etc.). You can have three bricks, and your friend has five bricks. They also have a concept for ratios, so you have 3/5ths as many bricks as your friend.

But in their system, you canât mix the two. You canât have 3 and 3/4 bricks, because fractions are only for ratios. In their system, having a fractional brick doesnât make sense, any more than saying you are six feet nine gallons tall in ours. Those units donât play well together. Mind you, their system is self-consistent and logical, but Iâd argue it has limited use. Fractions can be wildly multipurpose.

Itâs similar to infinite series. In the method you learned in high school, the series

1+2+3+4+5+â¦

doesnât converge and tends to go to infinity. That is also self-consistent, logical, but of limited use in this case. The rules of how we deal with series donât let you do much with that.

But there is a method called analytic continuation that does. It redefines things a bit, uses different rules that allow for dealing with such things. The mathematicians Euler and Riemann used it to get around the problems of infinite diverging series, and it allowed them to assign the value -1/12 to it. Those rules are self-consistent, logical, and highly useful. In fact, as I pointed out in the previous post, theyâre used to great success in many fields of physics. It gets complicated quickly, but you can read more about this here and especially here (that second one deals with this problem specifically, and in fact shows how analytic continuation can handle the problems of all the series presented in the Numberphile video). One of the greatest mathematicians the world has ever seen, Ramanujan, also did this. In fact, you should read about him; his story is as fascinating as it is tragic.

Anyway, neither set of rules is wrong. One is just better at handling certain things than the other. And you have to be sure to color within the lines depending on which rules you use. Which brings me to â¦

2) Canceled Series

Before I get to this, I want to say that early on in the main Numberphile video (and in my post) there is a link to the rigorous analytic continuation solution to this problem. So that part was good. However, they then employ a trick that is a bit of a no-no.

There are rules for dealing with infinite series, many developed by Cauchy in the 1800s. One of them is that when you have a series that diverges, that is, does not approach a finite limit, you canât go around adding and subtracting other series from it, or substituting values for it.

But in the video, they do just that. They write down Grandiâs series, show thatâs equal to ½ (more on that below), then use it to show that

1â2+3â4+5â¦

has ¼ as a solution. But given the rules of dealing with series in this way, thatâs a fudge (ironically, similar to the trick of âprovingâ 1=0, something I mentioned in my first post). So in the video where they multiply through the series, shift them, subtract them from one another ⦠thatâs not allowed. It works for a finite number of terms but leaves that aggravating tail of infinite terms to mess things up. That tail winds up wagging the dog, negating the whole thing.

Again, using Riemannâs and Eulerâs work, you can work this through legally. But using series written out in the way of the video, not so much.

3) Reaching My Limit

This may be of more interest to writers than math people. Caveat emptor.

Overall, a lot of what I wrote in the article is correct prima facie. A lot of it wasnât. How this came to be makes me a bit red-faced as well as has me chuckling at myself.

I did talk a bit about the analytic continuation method, called it rigorous, and said it shows that the series can have a value of -1/12. But I made a couple of mistakes: One was not trusting my instincts, and the other was trusting them too much.

In the first caseâand this is killing meâis that in my original draft of the post, I had a section pointing out you canât just add and subtract divergent series from each other! It was literally the first thing I wrote down after watching the video, because my math/science instinct told me there was a problem there. But I wound up removing it. Why?

Because of my writing instincts. I started digging into the cool Riemann stuff, and realized that since there really was a way to assign a value to the series, I didnât need to worry about the actual way they did it in the video. It was a trick, but it got the value I expected, so I took the section out. It made some sense at the time; I had the analytic stuff first, and the video second. I figured I had established the -1/12 bit, and it was good.

But the article wasnât working. I needed to rearrange it, put the video near the top of the post; starting it off with lots of thin-air math might not be the best bet. So I put the rigorous math after the video and totally left out the deleted section on the trick. Had I left it in, I suspect the new arrangement wouldâve triggered alarm bells in my head, and I wouldnât have been so laissez-faire with the video. Still, it didnât, and I wound up not dissecting something I should have.

On top of that, I shouldâve stressed the analytic solution more. I also should have stressed the idea that the examples I put in (the zigzag graph and the staircase) were only there as thought experiments to help understand the problem; they werenât meant to be rigorous. I probably should have just left that whole part out. Again, mea culpa.

Itâs an interesting balancing act, this writing about science and math. Sometimes it tips the wrong way. I blew it, and I'll try to be more careful in the future.

Term Limits

One more bit of exposition: You may have noticed that all through this post, I have avoided writing âThis series equals -1/12,â or âthe value of the sum of the series is -1/12.â This is due to my conversation with Ellenberg, which was fascinating to me. We talked about different methods, different rules, how new concepts were not accepted at first, and that things we think are simple now (like using fractions) were at one point in history heatedly debated as to their reality and usefulness. He put it very well:

It's not quite right to describe what the video does as âprovingâ that 1 + 2 + 3 + 4 + .... = -1/12. When we ask âwhat is the value of the infinite sum,â we've made a mistake before we even answer! Infinite sums don't have values until we assign them a value, and there are different protocols for doing that. We should be asking not what IS the value, but what should we define the value to be? There are different protocols, each with their own strengths and weaknesses. The protocol you learn in calculus class, involving limits, would decline to assign any value at all to the sum in the video.  A different protocol assigns it the value -1/12. Neither answer is more correct than the other.

Nice. Though Iâll add that one answer has more use than the other in certain circumstancesâthe point I made above.

This conversation led down the rabbit hole of how we use math and what for, and has inspired me to do some follow-up reading, more about the philosophy and development of various mathematical methods than the methods themselves. This is pretty cool stuff.

Other Methods

Finally, I got a lot of polite and informative notes from folks correcting me and pointing out details of all this, many of which overlapped with each other (including, interestingly, the last bit about the value assigned to a sum). Thanks to everyone who did so. Here are a few links I was sent for those who want to venture a few more terms down the series:

Ron Garretâs page on manipulating series
Colin Groveâs page
If you want some hairy details, Terence Tao has âem.
Bryden Cais goes through the steps with a clear (though technical) explanation
Just to be sure they get seen again: John Baezâs page on the Euler method, and Riemannâs as well

So: I made some mistakes, got other stuff right, couldâve been more clear, and learned a lot. Pretty much a typical day in anyoneâs book.

^*Why yes, I did just squeeze an appropriate math pun into a hip reference.